"$triranges" 修訂間的差異
(已建立頁面,內容為 "$triranges reading in the geometric parameters. $triregionnumber, and $triregiontype should be defined before the $triranges $triranges @f1 @f2 @f3 @f4...") |
|||
| (未顯示同一使用者於中間所作的 3 次修訂) | |||
| 行 25: | 行 25: | ||
[[檔案:pic1.png|700px|left]] |
[[檔案:pic1.png|700px|left]] |
||
| ⚫ | |||
| ⚫ | |||
| + | <br style="clear:both;"> |
||
Type5~8 are sine shape period structure. <br /> |
Type5~8 are sine shape period structure. <br /> |
||
| 行 44: | 行 45: | ||
[[檔案:pic2.png|700px|left]] |
[[檔案:pic2.png|700px|left]] |
||
| + | <br style="clear:both;"> |
||
| + | <br><br> |
||
| + | ---- |
||
<big><big>'''Example'''</big></big> |
<big><big>'''Example'''</big></big> |
||
| 行 64: | 行 68: | ||
10.0 12.0 2.0 0.0 0.0 0.0 |
10.0 12.0 2.0 0.0 0.0 0.0 |
||
13.0 4.0 14.0 5.0 0.0 0.0 |
13.0 4.0 14.0 5.0 0.0 0.0 |
||
| + | |||
| + | |||
| + | [[檔案:Trirange-1.png| 900px]] |
||
於 2024年8月22日 (四) 14:58 的最新修訂
$triranges reading in the geometric parameters. $triregionnumber, and $triregiontype should be defined before the $triranges
$triranges @f1 @f2 @f3 @f4 @f5 @f6 @f1 @f2 @f3 @f4 @f5 @f6
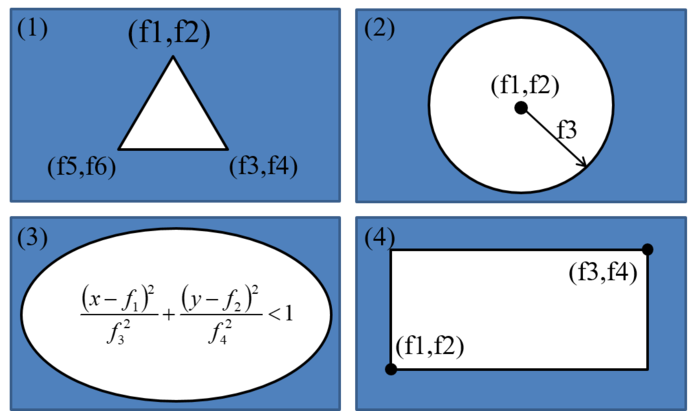
@f1 @f2 @f3 @f4 @f5 @f6 are defined below according to its type.
Example of @type
@type.1~4 are triangle, circle, ellipse and rectangle.
@type = 1 is triangle. If it have n-trianges, it it repeating along x direction.
@type = 11 is triangle. If it have n-trianges, it it repeating along y direction.
@type = 2 is circle. If it have n-circles, it it repeating along x direction.
@type = 21 is circle. If it have n-circles, it it repeating along y direction.
@type = 3 is ellipse. If it have n-ellipses, it it repeating along x direction.
@type = 31 is ellipse. If it have n-ellipses, it it repeating along y direction.
@type = 4 is rectangle. If it have n-rectangle, it it repeating along x direction.
@type = 41 is rectangle. If it have n-rectangle, it it repeating along y direction.
If the parameters are not show in the figure, you should set those parameters as 0.
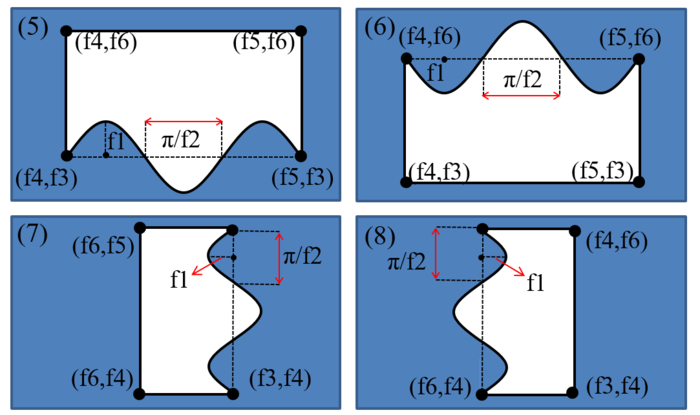
Type5~8 are sine shape period structure.
Type.5 and 6 can be determined by this expression:
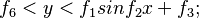
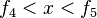
Type.7 and 8 can be determined by this expression:
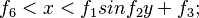
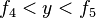
Example
For example:
$triregionnumber 3
$triregiontype 1 1 0.0 2 2 20.0 41 3 2.0
$triranges 4.0 4.0 8.0 4.0 6.0 8.0 10.0 12.0 2.0 0.0 0.0 0.0 13.0 4.0 14.0 5.0 0.0 0.0