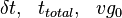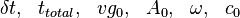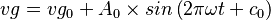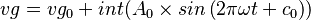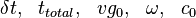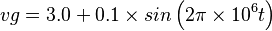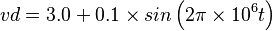"Solvetimestep2D" 修訂間的差異
出自 DDCC TCAD TOOL Manual
| (未顯示由 1 位使用者於中間所作的 2 次修訂) | |||
| 行 34： | 行 34： | ||
Steptype = 5: <br> |
Steptype = 5: <br> |
||
<math>\delta t,~~ t_{total},~~ vg_0 ,~~ \omega ,~~ c_{0}</math><br> |
<math>\delta t,~~ t_{total},~~ vg_0 ,~~ \omega ,~~ c_{0}</math><br> |
||
| − | <math>vg=vg_{end}</math> for t<0, for t>0, vg=<math> vg_0 </math> , generation = gen(system)*<math> (0.5+0.5*cos (\ |
+ | <math>vg=vg_{end}</math> for t<0, for t>0, vg=<math> vg_0 </math> , generation = system generation at t<0 generation = gen(system)*<math> (0.5+0.5*cos (2\pi \omega t + c_{0})) </math> <br> |
Steptype = 6: <br> |
Steptype = 6: <br> |
||
<math>\delta t,~~ t_{total},~~ vg_0 ,~~ \omega ,~~ c_{0}</math><br> |
<math>\delta t,~~ t_{total},~~ vg_0 ,~~ \omega ,~~ c_{0}</math><br> |
||
| − | <math>vg=vg_{end}</math> for t<0, for t>0, vg=<math> vg_0 </math> , generation = gen(system)*<math> Int(0.5+0.5*cos (\ |
+ | <math>vg=vg_{end}</math> for t<0, for t>0, vg=<math> vg_0 </math> , generation = system generation at t<0 generation = gen(system)*<math> Int(0.5+0.5*cos (2\pi \omega t + c_{0})) </math> <br> |
| + | |||
| + | Steptype = 7: <br> |
||
| + | <math>\delta t,~~ t_{total},~~ vg_0 ,~~ \omega ,~~ c_{0}</math><br> |
||
| + | <math>vg=vg_{end}</math> for t<0, for t>0, vg=<math> vg_0 </math> , generation = 0 at t<0 (Force Steady state to be 0), |
||
| + | generation = gen(system)*<math> (0.5+0.5*cos (2\pi \omega t + c_{0})) </math> <br> |
||
| + | |||
| + | Steptype = 8: <br> |
||
| + | <math>\delta t,~~ t_{total},~~ vg_0 ,~~ \omega ,~~ c_{0}</math><br> |
||
| + | <math>vg=vg_{end}</math> for t<0, for t>0, vg=<math> vg_0 </math> , generation = 0 at t<0 (Force Steady state to be 0), |
||
| + | generation =gen(system)*<math> Int(0.5+0.5*cos (2\pi \omega t + c_{0})) </math> <br> |
||
| 行 50： | 行 50： | ||
<math> vg=3.0 + 0.1 \times sin\left( 2\pi \times 10^{6} t \right) </math><br> |
<math> vg=3.0 + 0.1 \times sin\left( 2\pi \times 10^{6} t \right) </math><br> |
||
<math> vd=3.0 + 0.1 \times sin\left( 2\pi \times 10^{6} t \right) </math><br> |
<math> vd=3.0 + 0.1 \times sin\left( 2\pi \times 10^{6} t \right) </math><br> |
||
| + | |||
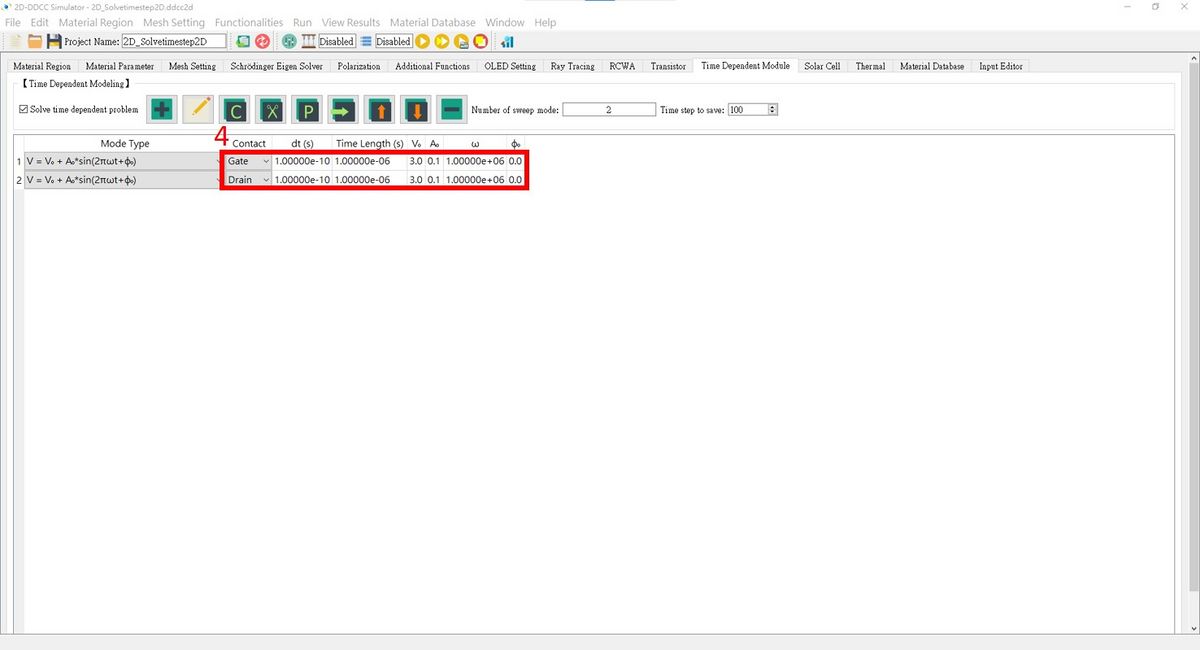
| + | <br>'''<big><big>The $Solvetimestep2D setting in GUI interface is here</big></big>''' <br> |
||
| + | 1. Press '''Time Dependent module''', check the box and press '''Add new sweep modes'''.<br> |
||
| + | 2. Fill in the fields as needed!<br> |
||
| + | [[檔案:2D_Solvetimestep2D_fig1.jpg|1200px]]<br> |
||
| + | [[檔案:2D_Solvetimestep2D_fig2.jpg|1200px]]<br> |
||
於 2024年11月20日 (三) 19:43 的最新修訂
$solvetimestep2D is a command for solving the transient behavior of the device. The format is
$solvetimestep2D number_of_different_steps(Nt) steptype contact_typepar1 par2 par3 par4 .... steptype contact_type
The number of parameters depeding on step type. Now we have 3 step types
Steptype = 1:
for t<0, for t>0, vg=
Steptype = 2:
Steptype = 3:
contact_type
2: gate 3: source 4: drain
Steptype = 4:
, generation = system generation at t<0 and generation =0 for t> 0
Steptype = 5:
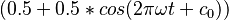
Steptype = 6:
Steptype = 7:
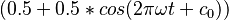
Steptype = 8:
For example:
$solvetimestep2D 2 2 2 1.0e-10 1.0e-6 3.00 0.1 1.0e6 0.0 2 4 1.0e-10 1.0e-6 3.00 0.1 1.0e6 0.0
The $Solvetimestep2D setting in GUI interface is here
1. Press Time Dependent module, check the box and press Add new sweep modes.
2. Fill in the fields as needed!
related:
$savetimestep2D
