$DoEalloyfluc 2D
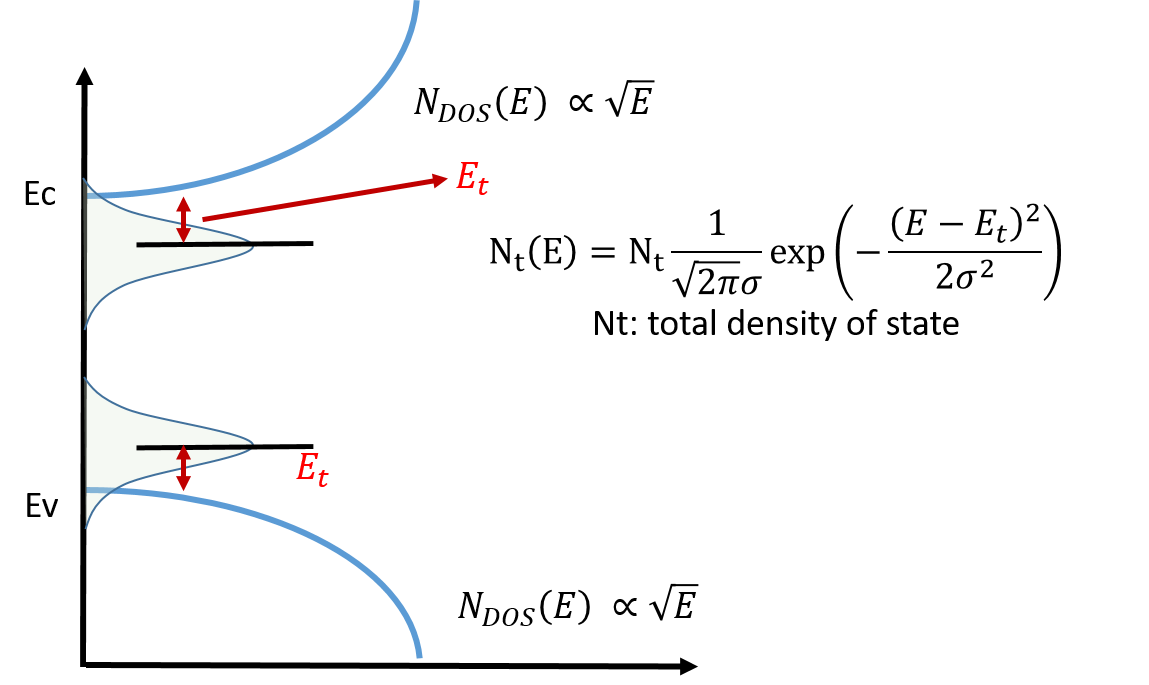
Set Gaussian-like density of state. This model usually use for organic material or energy disorder material such as some leakage path for InGaN/GaN QB. It can also be used in amorphous Si, etc..
Format
- Type 11 is electron only
- Type 12 is for electron only but with 2 states
$DoEalloyfluc 11 Nt Et σ
$DoEalloyfluc 12 Nt1 Et1 σ2 Nt2 Et2 σ2
If the Gaussian peak level is above conduction band, then Et is positive. If the Gaussian peak value is below Ec, then the value is negative.
Et is defined as Et = Et - Ec
- Type 21 is hole only
- Type 22 is hole only but with 2 states
$DoEalloyfluc 21 Nt Eth σ
$DoEalloyfluc 22 Nt1 Eth1 σ1 Nt2 Eth2 σ2
If the Gaussian peak level is above valence band, then Et is positive. If the Gaussian peak value is below Ev, then the value is negative.
Eth is defined as Eth = Eth - Ev
- Type 31 is electron and hole with 2 gaussian shape. The first one is for electron and the second one is for hole.
$DoEalloyfluc 31 Nt_e Et_e σ_e Nt_h Et_h σ_h
Where e means electron properties of DOS in this layer.
- Type 32 is electron and hole both with 2 gaussian shape. The first 2 are for electron and the second two are for hole.
$DoEalloyfluc 32 Nt1_e Et1_eσ_e Nt1_e Et2_e σ2_e Nt1_h Et1_h σ1_h Nt2_h Et2_h σ2_h
Where e1 means electron properties of DOS1 and h2 means hole properties of DOS2.
Parameter Explanation
Nt : Carrier concerntration. 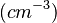
Et : Depth of dos central and Ec/Ev. 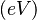
sigma : FWHM of the gaussian distribution. 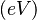
- Type 41 is for 2D density of states. The density of state is like a step function. Et_e is the position related to Ec. Et_hh and Et_lh are the position related to Ev.
$DoEalloyfluc 41 Et_e Et_hh Et_lh m_e_parallel me_e_perp m_hh m_lh film_thickness(cm)
Film_thickness(cm) : For 2D material, the carrier density is decided by m/pi/hbar^2 * ln(1+exp((Efn-En)/KBT)). But the unit is 1/cm^2. For simulation, we need to convert it into unit of 1/cm^3. For MoS2 liked material, the thickness could be 6A (Film thickness). For approaching the density of state in QW (may not be suitable), the film_thickness(cm) could be QW's thickness. I don't suggest to use this for QW. This is designed for 2D materials.
- Type 45 is for linear density of states. The density of state is like a Dirac cone.
The density of state for electrons can be expressed as E= a_e*k. Usually a_hh=a_e (for graphene). If this function is used a_hh is equal to HH hole. If LH is not going to be included, LH=0 or very small value. Note thatfor boltzmann approximation, Therefore, a_e cannot be zero.
$DoEalloyfluc 45 a_e a_hh film_thickness(cm)
Film_thickness(cm) : For 2D material, the carrier density of state is 1/cm^2. For simulation, we need to convert it into unit of 1/cm^3. This is designed for graphene like 2D materials.